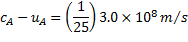Judgement Day (22 page)

The passenger (A) sees
the light pulse travelling in a perfectly vertical direction. The
observer (B) sees the light pulse follow a diagonal path. Since the
observer (B) experiences a longer time interval than the passenger
whilst observing the light pulse cover a greater distance than the
passenger (A), light speed appears constant for both passenger (A)
and observer (B).

For simplicity of
numbers I shall use a train travelling at a velocity ‘u’ of 3/5 of
the speed of light. Using the Lorentz transforms, the mathematics
are as follows. [The subscript ‘A’ denotes time and distance as
measured by the passenger, no subscript denotes time and distance
as measured by the relatively stationary observer.]

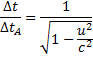
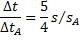
The speed of light for
the passenger is given by

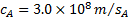
A conversion of units
yields

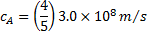
Adding vertical
velocity of light to horizontal velocity of the train by Pythagoras
yields

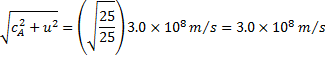
Therefore the light
pulse appears to travel at a constant 3x10
⁸
m/s for both
the passenger (A) and the relatively stationary observer (B). While
this thought experiment is very commonly found in textbooks, the
mathematics does not work when the light pulse is travelling in any
direction other than the vertical (from the perspective of the
passenger (A)). [Figure 4b]

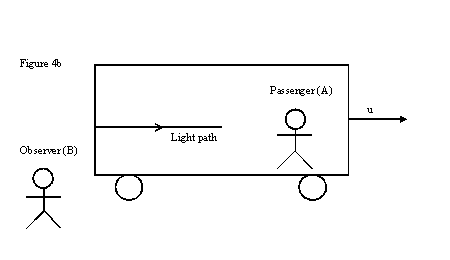
In the next example,
as shown in the diagram above, both the passenger (A) and the
relatively stationary observer (B) see the light pulse travel in
the same direction as the train. For simplicity of numbers I shall
again use a train travelling at a velocity ‘u’ of 3/5 of the speed
of light. For this example length ‘l’ dilation also needs to be
taken into consideration.

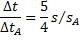
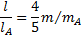
The speed of light for
the passenger on the train is again given by

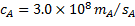
A conversion of units
yields

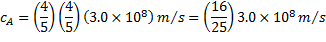
Adding velocity of
light to velocity of train gives

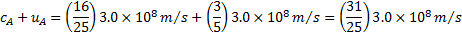
Which is >
3.0x10
⁸
m/s and, skipping the calculations, if the pulse
of light travels in the opposite direction to the train then the
speed of the light pulse for the outside observer (B) is