The Cosmic Landscape (48 page)

Let’s take a look inside one of the bubbles. What do we find? Typically we will find ourselves located in a valley with altitude somewhat lower than at the start. Space inside the bubble will also be inflating. I’m not speaking about the ordinary growth of the bubble but about the cloning of space inside the bubble. Thus, we start over again. A new patch of space is now located in a new valley. But there are still other, lower valleys. Inside the original bubble, a next-generation bubble may form in another nearby valley with a lower altitude. And if that bubble is bigger than critical size, it begins to grow—a growing bubble inside another bubble.
As a rule, I don’t like biological analogies in physics. People tend to take them too literally. I’m going to use one now but
please
don’t get the idea that I think that universes or black holes or electrons are alive, engage in Darwinian competition, or have sex.
Think of the megaverse as a colony of organisms that reproduce by cloning. In order to avoid confusion, let me emphasize: the organisms are not living creatures—they are reproducing regions of space. Because clones are identical to their parents, we can think of them as occupying the same valley in the Landscape. We might even think of a Landscape of biological designs: different valleys corresponding to different species. Don’t worry about the organisms getting in each other’s way. In this fictitious world there is always room for more. When a bubble forms with different properties from the parent, the offspring occupies a new neighboring valley. Because the space inside the bubble also inflates, the offspring begins both the cloning process and the process of inhabiting new valleys by creating next-generation bubbles. In this way the metaphorical colony begins to spread out over the Landscape. The fastest reproducers are the patches of space at the highest altitude where the cosmological constant is largest. In these regions of the Landscape, cloning takes place especially rapidly, and the population of the high altitudes grows fastest. But the high-altitude organisms also feed the lower altitudes so that the population in the nether regions also grows with time.
4
Eventually every niche in the Landscape will become populated, not just once but with an exponentially increasing population. The only thing wrong with the analogy is that real organisms compete and kill each other when their valley becomes overcrowded. No mechanism for competition between pocket universes exists, so the population of every valley continues to increase indefinitely. One might think of these organisms as being totally invisible to one another in every way.
How do bubbles die? If a bubble appears with exactly zero cosmological constant, it will fail to inflate and cease reproducing. The only such vacuums are the supersymmetric parts of the Landscape. Thus, the supersymmetric region of the Landscape is the graveyard of universes in more than one sense. Ordinary life cannot exist in supersymmetric environments, but more to the point, reproduction of bubbles ceases.
While analogies often capture some truth in a way that is easy to understand, they are always misleading in other ways. The analogy between Eternal Inflation and species evolution is defective in ways other than the lack of competition. Darwinian evolution depends on continuity between generations. Offspring closely resemble their parents. If we had a sequence of photographs of all the generations of apes, beginning with the “missing link” five million years ago and ending with you or me, we could line them up in a row to see how rapidly evolution did its work. If we ignore those changes that distinguish individuals at a given time, we would find that the changes from one generation to another are far too tiny to detect. Only the accumulated change over thousands of generations would be noticeable, and then just barely. The same would be true of all types of life. Big structural changes take place very infrequently, and when they do they almost always lead to evolutionary dead ends. Anyone born with two heads, three legs, or no kidneys won’t survive for more than a short time (except in modern-day hospitals), and in any case, such creatures are extremely unlikely to be successful in the Darwinian dating game.
The contrast with evolution on the cosmic Landscape could not be bigger. The change that takes place when a bubble nucleates in an inflating region of space is not incremental like biological evolution. Think geologically: neighboring valleys are dissimilar. Aspen valley, in the Colorado Rockies, at altitude eight thousand feet, is more than two thousand feet lower than Twin Lakes, just on the other side of In-dependence Pass. They are dissimilar in other ways as well. If there is a valley so similar to Aspen that we would hardly notice the difference, chances are it’s a long way off.
That’s the way the cosmic Landscape is. The altitude of neighboring valleys is not particularly similar. If the neighbors differ in the composition of branes or fluxes, it will lead to differences in the list of elementary particles, the constants of nature, and even the dimensionality of space. When a parent vacuum gives birth to an offspring bubble, the results typically will be a monstrous mutation rather than a small incremental change.
Is Eternal Inflation, with its wildly prolific creation of bubble-worlds of every possible kind, a wild phantasmagoric hallucination? I don’t think so. The exponential expansion of space seems rock solid; no cosmologist questions it. The possibility of more than one valley is in no way unusual, nor is the assumption that an inflating region will spin off bubbles of lower altitude. Everyone agrees.
What is new is that String Theory gives rise to an exponentially large number of valleys with a tremendous variety of environments. Many physicists are very alarmed by this idea. But even here, most serious string theorists admit that the reasoning looks solid.
5
Let’s consider the last stages of cosmic evolution just before our patch settles into the conventional era of Inflation followed by reheating and eventually life. Where did we come from before miraculously appearing on the inflationary ledge? Most likely the answer is a neighboring valley at a higher elevation. How does that valley differ from our own? String Theory gives answers: fluxes had other values, branes were in different locations, and the moduli of the compactification were different. Perhaps in the passage over the mountain toward the ledge, branes annihilated one another and rearranged, fluxes shifted, and the sizes and shapes of several hundred moduli changed to something new to give a new Rube Goldberg machine. And with the new arrangement came new Laws of Physics.
Einstein’s General Theory of Relativity can lead to consequences that defy our usual abilities to visualize geometric relations, black holes being a prime example. Another extremely interesting curiosity involves the geometry inside bubbles that form in an inflating space. From the outside the bubble appears to be an expanding sphere bounded by a domain wall or membrane. The energy that is released by the changes inside the bubble is converted to kinetic energy of the domain wall, which quickly accelerates. After a short time the bubble will be expanding with almost the speed of light. One would expect that an observer inside the bubble would experience a finite world that at every instant is bounded by a growing wall. But that’s not at all what he sees. The view from inside the bubble is very surprising.
In chapter 5 we encountered the three basic kinds of expanding universes: the closed-and-bounded universe of Alexander Friedmann, the flat universe, and the infinite open universe with negative curvature. All of the standard universes are homogeneous, and none of them has an edge or wall. One might think that an inhabitant in the interior of a bubble would observe the expanding domain wall and conclude that he didn’t live in any of the standard universes. Surprisingly, this is incorrect: that inhabitant of the bubble would observe an
infinite
open universe with negatively curved space! How a finite expanding bubble can look like an infinite universe from the inside is one of those mysterious paradoxes of non-Euclidean Einsteinian geometry.
I will try to give you some idea of how the paradox is resolved. Let’s start with a map of the earth. Because the earth’s surface is curved, it cannot be drawn on a flat plane without distortion. For example, in a Mercator projection, Greenland looks almost as big as North America and a good deal bigger than South America and Africa. Of course it is nowhere near as big as these continents. But to flatten the earth’s surface, a great deal of stretching is necessary.
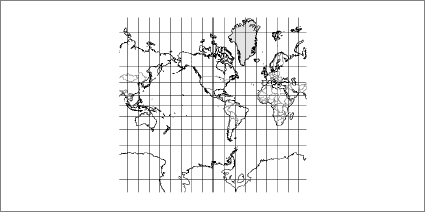
The same is true if we try to flatten a negatively curved surface so that it can be drawn on a plane. It’s not easy to draw such a space, but fortunately, a famous artist has already done the work. The artist M. C. Escher’s famous woodcut
Limit Circle IV
is nothing but a uniformly negatively curved space drawn on a flat piece of paper. All the angels are the same size, and so are all the devils. They can roughly be thought of as galaxies. But to flatten the space, the center has to be stretched, and the distant parts must be compressed.
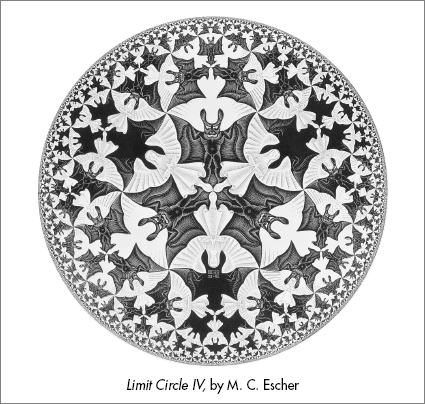
In fact the distance from the center of the space to the boundary is infinite. An infinite number of devils (or angels) must be crossed in order to get to the edge. Because each devil is the same size as all the others, the distance is also infinite. Nevertheless, the whole infinite space appears as the interior of a circle when it is flattened onto a plane. With this in mind it is not so difficult to picture the infinite geometry fitting into a finite bubble.
What is especially strange is that if the astronomer wanted to study the expanding domain wall, he would always find it infinitely far away. Interior to the bubble, the geometry of space is unbounded, despite the fact that at any moment of time an exterior observer sees the bubble as a bounded sphere. It’s not that an astronomer inside the bubble can’t detect light coming from the domain wall. But that light does not seem to be coming from a boundary of space; rather, it seems to come from a boundary of time—from what appears to be a Big Bang taking place in the past. This is a most paradoxical situation, an infinite expanding universe inside a finite expanding bubble.
