Antifragile: Things That Gain from Disorder (52 page)
Read Antifragile: Things That Gain from Disorder Online
Authors: Nassim Nicholas Taleb

How to punish with a stone—I landed early (once)—Why attics are always useful—On the great benefits of avoiding Heathrow unless you have a guitar

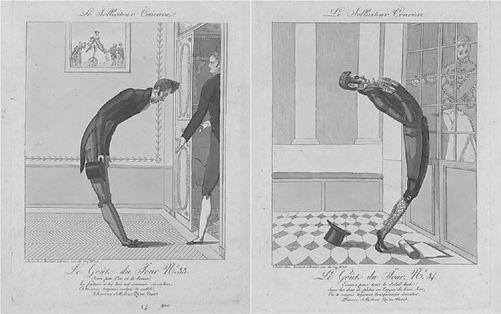
FIGURE 8.
The solicitor knocking on doors in concave (left) and convex (right) position. He illustrates the two forms of nonlinearity; if he were “linear” he would be upright, standing straight. This chapter will show—a refinement of Seneca’s asymmetry—how one position (the convex) represents antifragility in all its forms, the other, fragility (the concave), and how we can easily detect and even measure fragility by evaluating how humped (convex) or how slumped (concave) the courtier is standing.
I noticed looking at the porcelain cup that it did not like volatility or variability or action. It just wanted calm and to be left alone in the tranquility of the home study-library. The realization that fragility was simply
vulnerability to the volatility of the things that affect it
was a huge personal embarrassment for me, since my specialty was the link between volatility and nonlinearity; I know, I know, a very strange specialty. So let us start with the result.
A story present in the rabbinical literature (
Midrash Tehillim
), probably originating from earlier Near Eastern lore, says the following. A king, angry at his son, swore that he would crush him with a large stone. After he calmed down, he realized he was in trouble, as a king who breaks his oath is unfit to rule. His sage advisor came up with a solution. Have the stone cut into very small pebbles, and have the mischievous son pelted with them.
The difference between a thousand pebbles and a large stone of equivalent weight is a potent illustration of how fragility stems from nonlinear effects. Nonlinear? Once again, “nonlinear” means that the response is not straightforward and not a straight line, so if you double, say, the dose, you get a lot more or a lot less than double the effect—if I throw at someone’s head a ten-pound stone, it will cause more than twice the harm of a five-pound stone, more than five times the harm of a one-pound stone, etc. It is simple: if you draw a line on a graph, with harm on the vertical axis and the size of the stone on the horizontal axis, it will be curved, not a straight line. That is a refinement of asymmetry.
Now the very simple point, in fact, that allows for a detection of fragility:
For the fragile, shocks bring higher harm as their intensity increases (up to a certain level).
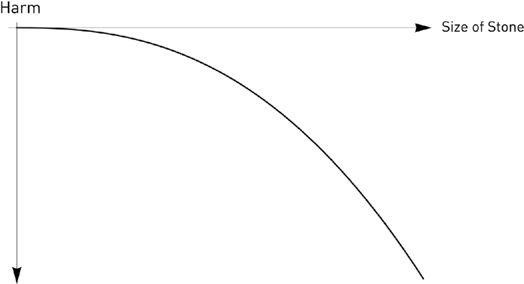
FIGURE 9
. The King and His Son. The harm from the size of the stone as a function of the size of the stone (up to a point). Every additional weight of the stone harms more than the previous one. You see nonlinearity (the harm curves inward, with a steeper and steeper vertical slope).
The example is shown in
Figure 9
. Let us generalize. Your car is fragile. If you drive it into the wall at 50 miles per hour, it would cause more damage than if you drove it into the same wall ten times at 5 mph. The harm at 50 mph is more than ten times the harm at 5 mph.
Other examples. Drinking seven bottles of wine (Bordeaux) in one sitting, then purified water with lemon twist for the remaining six days is more harmful than drinking one bottle of wine a day for seven days (spread out in two glasses per meal). Every additional glass of wine harms you more than the preceding one, hence your system is fragile to alcoholic consumption. Letting a porcelain cup drop on the floor from a height of one foot (about thirty centimeters) is worse than twelve times the damage from a drop from a height of one inch (two and a half centimeters).
Jumping from a height of thirty feet (ten meters) brings more than ten times the harm of jumping from a height of three feet (one meter)—actually, thirty feet seems to be the cutoff point for death from free fall.
Note that this is a simple expansion of the foundational asymmetry we saw two chapters ago, as we used Seneca’s thinking as a pretext to talk about nonlinearity. Asymmetry is necessarily nonlinearity. More harm than benefits: simply, an increase in intensity brings more harm than a corresponding decrease offers benefits.
Let me explain the central argument—why fragility is generally in the nonlinear and not in the linear. That was the intuition from the porcelain cup. The answer has to do with the structure of survival probabilities: conditional on something being unharmed (or having survived), then it is more harmed by a single rock than a thousand pebbles, that is, by a single large infrequent event than by the cumulative effect of smaller shocks.
If for a human, jumping one millimeter (an impact of small force) caused an exact linear fraction of the damage of, say, jumping to the ground from thirty feet, then the person would already be dead from cumulative harm. Actually a simple computation shows that he would have expired within hours from touching objects or pacing in his living room, given the multitude of such stressors and their total effect. The fragility that comes from linearity is immediately visible, so we rule it out because the object would be already broken. This leaves us with the following: what is fragile is something that is both unbroken and subjected to nonlinear effects—and extreme, rare events, since impacts of large size (or high speed) are rarer than ones of small size (and slow speed).
Let me rephrase this idea in connection with Black Swans and extreme events. There are a lot more ordinary events than extreme events. In the financial markets, there are at least ten thousand times more events of 0.1 percent magnitude than events of 10 percent magnitude. There are close to eight thousand microearthquakes daily on planet Earth, that is, those below 2 on the Richter scale—about three million a year. These are totally harmless, and, with three million per year, you would need them to be so. But shocks of intensity 6 and higher on the scale make the newspapers. Take objects such as porcelain cups. They get a lot of hits, a million more hits of, say, one hundredth of a pound per square inch (to take an arbitrary measure) than hits of a hundred pounds per square inch. Accordingly, we are necessarily immune to the
cumulative
effect of small deviations, or shocks of very small magnitude, which implies that these affect us disproportionally less (that is, nonlinearly less) than larger ones.
Let me reexpress my previous rule:
For the fragile, the cumulative effect of small shocks is smaller than the single effect of an equivalent single large shock.
This leaves me with the principle that the fragile is what is hurt a lot more by extreme events than by a succession of intermediate ones. Finito—and there is
no other
way to be fragile.
Now let us flip the argument and consider the antifragile. Antifragility, too, is grounded in nonlinearties, nonlinear responses.
For the antifragile, shocks bring more benefits (equivalently, less harm) as their intensity increases (up to a point).
A simple case—known heuristically by weight lifters. In the bodyguard-emulating story in
Chapter 2
, I focused only on the maximum I could do. Lifting one hundred pounds once brings more benefits than lifting fifty pounds twice, and certainly a lot more than lifting one pound a hundred times. Benefits here are in weight-lifter terms: strengthening the body, muscle mass, and bar-fight looks rather than resistance and the ability to run a marathon. The second fifty pounds play a larger role, hence the nonlinear (that is, we will see,
convexity
) effect. Every additional pound brings more benefits, until one gets close to the limit, what weight lifters call “failure.”
1
For now, note the reach of this simple curve: it affects about just anything in sight, even medical error, government size, innovation—anything that touches uncertainty. And it helps put the “plumbing” behind the statements on size and concentration in
Book II
.
Nonlinearity comes in two kinds: concave (curves inward), as in the case of the king and the stone, or its opposite, convex (curves outward). And of course, mixed, with concave and convex sections.
Figures 10
and
11
show the following simplifications of nonlinearity: the convex and the concave resemble a smile and a frown, respectively.
FIGURE 10
. The two types of nonlinearities, the convex (left) and the concave (right). The convex curves outward, the concave inward.
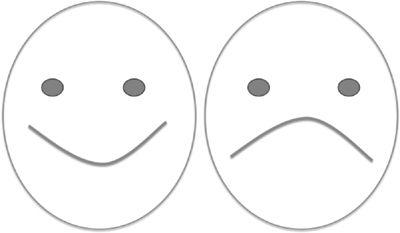
FIGURE 11.
Smile! A better way to understand convexity and concavity. What curves outward looks like a smile—what curves inward makes a sad face. The convex (left) is antifragile, the concave (right) is fragile (has negative convexity effects).