The Calendar (21 page)


These number symbols were a great improvement over stick-like signs, but still presented problems for calculating or recording complicated equations and large numbers. This is why a positional system was such a phenomenal breakthrough--a leap of inspiration made by a long-forgotten Mesopotamian who undoubtedly became frustrated with writing out large numbers. Perhaps he was a scribe assigned the unenviable task of counting barrels of wine coming and going from Ur’s royal palace. Or an architect designing a ziggurat but running out of space on a clay tablet as he made his calculations, and so he invented a quick shorthand to save space. Here is what 365 looks like in cuneiform with its positional notation:

It was this problem of unwieldiness--and more--that the Indians solved by inventing our system of nine numeric symbols sequenced in positional notation, later adding zero for the tenth symbol.
How exactly the Vedics of India figured out this brilliantly simple scheme is another mystery, though they might have been inspired to transform Mesopotamia’s base-60 positional system into their own base-10. Some historians also speculate that a connection exists between Indian numbers and ancient Chinese rod numerals, which also have symbols for 1 through 10 used--after the third century AD--in a positional system.
Whatever its origin, these symbols that eventually became our own first appear in carvings on stone columns across north India as early as 250 BC or before, when Hindu mathematics was making the transition to a positional system. Written out in the early Hindu script known as Brahmi, the first nine numbers look like this:
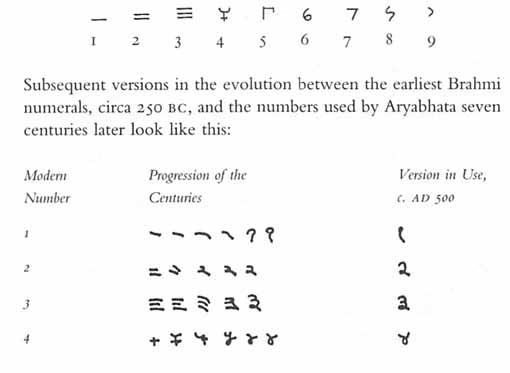

The evolution from this version of Brahmi to a ten-digit positional notation is not entirely clear. Historians suspect that the motivation to drop the Brahmi symbols beyond the number nine came from the demands of the Hindu religion, which uses a calendar encompassing enormous spans of time to date its creation myths. These form a religious chronology stretching back millions of years, requiring the manipulation of large numbers--which is far easier when one uses powers of ten. The Indian use of counting boards also encouraged the development of number symbols that were simple and few in number.
The timing is also uncertain. Aryabhata was certainly aware of positional notation and apparently used it in his day-to-day calculations. But because he wrote his treatises in metrical verse he used words and letters to represent numbers--the equivalent of us writing out ‘twenty-nine’ rather than 29--in an attempt at mathematics as poetry.
The first known use in India of the nine-digit positional system has been discovered on a plate and dated to the year 595. The number is a date--346--written in a decimal positional notation.
The first outside mention of the Hindus’ system of nine numbers comes in 662 from the Syrian Severus Sebokht, an academic and bishop who lived in a Greek community founded a century earlier by scholars fleeing Athens after Justinian closed Plato’s Academy, which he had accused of fostering paganism. Apparently Sebokht became piqued at his colleagues’ disdain for any knowledge beyond the Greek sphere. Writing about the Hindus, he cites their ‘subtle discoveries of astronomy . . . their valuable methods of calculation, and their computing that surpasses description. I wish only to say that this computation is done by means of nine signs’.
But nine does not make ten, meaning the system was not complete without zero, a concept critical to understanding the advanced mathematics needed to create an accurate calendar. Zero developed as Indians using the nine numbers for calculations found themselves needing to keep an empty column on their counting boards to represent ‘nothing’, an idea they transferred to writing out numbers by leaving a space. But this could be confusing, since a space could mean either an empty position in a single number or the space between two separate numbers. To avoid confusion somebody along the way decided to make something of ‘nothing’.
Who was first to scratch out a symbol for zero is yet another mystery. In Mesopotamia a symbol for the empty position appears late for this ancient civilization, arriving around the time of Alexander’s invasion or just after, represented by two small wedges placed obliquely:
0
At roughly the same time or shortly thereafter the Indians began using a dot, a symbol that became widespread enough by the sixth century that the Indian poet Subandhu used it as a metaphor in his poem Vasavadatta:
And at the time of the rising of the moon with its blackness of night, bowing low, as it were, with folded hands under the guise of closing the blue lotuses, immediately the stars shone forth . . . like zero dots . . . scattered in the sky as if on the ink blue skin rug of the Creator who reckoneth the total with a bit of moon for chalk.
The Indians referred to this ‘nothing’-dot as
sunya,
meaning void or empty. Our word
zero
comes from
sifr,
the Arabic version of
sunya,
which medieval Europeans altered to
ziphirum
in Latin.
Greeks of the classic age had no symbol for zero, because their numerical system did not require a zero place. But they were aware of the concept of a number that stood for nothing. Indeed, Aristotle rejected it as a non-number to be ignored, since one cannot divide by zero, or divide zero by itself. Nevertheless, Eurocentric scholars long assumed that the symbol for zero was invented by the Greeks, with no proof at all, speculating that it came from the Greek letter omicron--O--the first letter in the Greek word
oudeu,
meaning ‘empty’. But this unwarranted belief that Indians could not have come up with such a basic concept has given way to recognition that ancient Greeks did not really use such a symbol for zero, and that Indian mathematicians seem independently to have invented the dot and then the round goose-egg symbol. The first use of this symbol for zero in India appears in the year 876 in an inscription found in the Gwalior region south of Delhi, containing two numbers with zeros.
This comes two centuries after Severus Sebokht’s mention of the
nine
Hindu numbers, though archaeologists have found the round symbol for zero in two numbers in an inscription in Malaysia the numbers 60 and 606 that dates to AD 684. The Malay peninsula was then under Indian influence. Some historians also believe a treatise on mathematics known as the Bakhshali Manuscript may have been written as early as the third century AD. It contains numbers with zeros and a fully developed decimal place-value system. The numbers include:

The first use of zero as a fully formed number seems to have appeared around the time of Brahmagupta in the seventh century, when this great Indian mathematician tried, but failed, to explain how zero could be divided by itself. The Maya also invented a true zero in about the third century AD, using several symbols, including a half-open eye--Ɵ--which they used to indicate missing positions as they wrote out numbers to represent time intervals in their calendar.
This explanation of zero does not quite finish our story about the mathematics needed to correct the calendar, since the year is not 365 days long, but 365.242199 days, give or take a few seconds. In other words, we have this pesky fraction to contend with, expressed here as a
decimal
fraction. This concept--and the ease with which we are able to represent this value--also did not come easily or all at once. Beyond the simplest divisions of a whole number, fractions posed a huge problem for humanity through most of history.
How do you divide three sacks of grain among five people? And how do you split up a year, month, day, hour or minute into smaller parts?

Several modern words are derived from this system--for instance,
ounce
and
inch
come from
uncia.
But these symbols are far too cumbersome and imprecise for sophisticated values and calculations. For instance, it was relatively simple for a Roman--or Bede, or Alcuin in Charlemagne’s court to write out the Latin whole number and fraction for the length of Caesar’s year, which is CCCLXV
=-
days--365 1/4. But try writing the true solar year of 365.242199 days--the equivalent of 365 242,199/1,000,000--in Roman numerals. No symbol exists in Latin for such a precise number, a reality that profoundly affected the pursuit of determining an accurate year. Nor is it possible to calculate in Roman numerals a value that takes into account variations in the earth’s motions, including the gradual slowing of the tropical year over the centuries.
