The Cosmic Landscape (19 page)

Who’s to say that beauty of this type has less merit than the reductionist-particle physicist’s version? Not I. But the kind of beauty that I am speaking about is different. Elementary-particle physicists search for beauty in the underlying laws and equations. Most have had a kind of quasireligious belief in the gods of uniqueness and simplicity. As far as I can tell, they believe that at the “bottom of it all” lies a beautiful theory, a single unique powerful and compelling set of equations describing all phenomena, at least in principle, even if the equations are too hard to solve. These master equations should be simple and symmetric. Simplicity, roughly speaking, means it should be possible to write the equations in a box about this big:

But above all, the equations should uniquely predict the Laws of Physics that have been discovered over the last few centuries, including the Standard Model of particle physics: the list of elementary particles, their masses, coupling constants, and the forces among them. No other alternative rules should be possible.

The myth of uniqueness and elegance probably originated with our Greek intellectual forebears. Pythagoras and Euclid both believed in a mystical mathematical harmony of the universe. Pythagoras believed that the world operated according to mathematical principles that paralleled those governing music. While the connection between music and physics may seem to us naive and even silly, it’s not hard to see in the Pythagorean creed the same love of symmetry and simplicity that so motivates modern physicists.

Euclidean geometry also has a strong esthetic flavor to it. Proofs should be as simple and elegant as possible, and the number of unproved axioms should be as small as possible: no more than five of them are needed. Euclidean geometry is usually considered a branch of mathematics. But the Greeks made no distinction between mathematics and physics. For them, Euclidean geometry was the theory of how real physical space behaves. You could not only prove theorems, but you could also go out and measure the properties of space, and they would necessarily (according to the Greeks) agree with the theorems. For example, you may draw a triangle using a ruler and pencil and then with a protractor measure the three interior angles. One of the theorems of Euclidean geometry states that the sum of these angles is exactly 180 degrees. The Greeks believed that any real triangle, drawn in real space, would agree with the theorem. Thus, they were making statements about the physical world, statements they believed were not only true but also unique. Space, they believed, conforms to Euclid’s axioms, and moreover, it could be no other way. Or so they thought.

Plato and Aristotle went further, adding a particularly esthetic element to the laws of astronomy. To them the circle was the perfect figure. Every point being equally distant from the center, the circle has perfect symmetry; no other figure is as symmetric. So Plato, Aristotle, and their later followers believed that no other figure could control and regulate the motions of planets. They believed the heavens were composed of a set of elegant crystal shells, perfectly transparent, perfectly round, and moving with perfect clockwork precision. They could not conceive of it being any other way.

The Greeks had an equally elegant theory of terrestrial phenomena, which paralleled modern-day physicists’ hopes for a unified theory. They believed that all earthly matter was made of four elements: earth, air, water, and fire. Each had its appropriate place and tended to migrate toward that place. Fire was the lightest and, therefore, tended to rise. Earth, being the heaviest, tended to sink to the lowest elevation. Water and air were in between. Four elements and one dynamical principle: you would be surprised at how much they could explain. The only thing missing was uniqueness. I don’t see why there can’t be additional elements: earth, air, fire, water, red wine, cheese, and garlic.

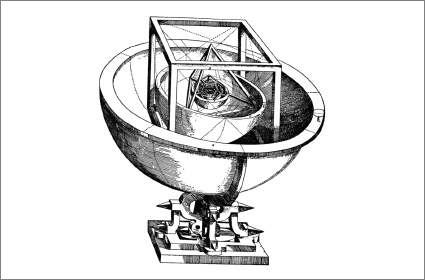
In any case, astronomers, alchemists, and chemists threw a wrench into the Greek schemes. Johannes Kepler knocked the circle from its high pedestal and replaced it with the more varied and less symmetrical elliptical planetary orbits. But Kepler, too, believed in Pythagorean mathematical harmony. At the time there were only five known planets: Venus, Mars, Jupiter, Saturn, and of course, Earth. Kepler was deeply impressed by the fact that there are exactly five regular polyhedra, five Platonic solids: the tetrahedron, octahedron, icosahedron, cube, and dodecahedron.
⁴
Kepler was seduced by what he saw as an inevitable and beautiful correspondence between the planets and the Platonic solids. And so he built a mathematical model of the universe as a set of nested polyhedra, with the goal of explaining the radii of their orbits. I’m not sure we would find it elegant, but he did, and that’s the important thing. Elegant or not, the five Platonic solids are unique. The theory, of course, was utter nonsense.

At the same time alchemists could not make progress without recognizing the need for more than four elements. By the end of the nineteenth century, they had identified almost one hundred elements, and nature was losing some of its simplicity. The periodic table put some order into chemistry, but it was a far cry from the simplicity and uniqueness that the Greeks had demanded.

But then, in the early twentieth century, Bohr, Heisenberg, and Schrödinger discovered the principles of quantum mechanics and atomic physics, thus providing a precise foundation for chemistry. The number of elements swung back to only four: not the Greek four, but the photon, electron, proton, and neutron. All of chemistry can be (in principle only) unambiguously derived from the quantum mechanical theory of these four elementary particles. Simplicity, elegance, and uniqueness were again gaining the upper hand. The basic principles of relativity, quantum mechanics, and the existence of the four elements would give rise to all chemical behaviors if only we had the power to solve the equations. This was very close to the physicist’s ideal.

But, alas, it was not to be. Elementary particles were discovered wholesale: neutrinos, muons, so-called strange particles, mesons, and hyperons, none of them having a place in the simple scheme of things. They played no important role in the description of matter, yet there they were, muddying the waters. Elementary-particle physics in the 1960s was a hopeless muddle of Greek and Latin letters denoting hundreds of supposed elementary particles. As a young physicist hoping to find beauty and elegance in the laws of nature, I found the whole mess depressing.

But then the 1970s improved the outlook. Quarks replaced the protons, neutrons, and mesons as the constituents of the nucleus, and—as discussed in chapter 1—the single quantum field theory called Quantum Chromodynamics (QCD) could explain everything about protons, neutrons, mesons, and nuclei and the less familiar, so-called strange particles (particles containing strange-quarks). The number of elements had decreased somewhat. At the same time electrons and neutrinos were understood to be twins related by a deep, underlying symmetry. The tug of war was again shifting to the advantage of simplicity. Finally, the Standard Model came into full existence in the mid-1970s, providing a complete description of all known phenomena (or so it is sometimes claimed), but in terms of about thirty arbitrary parameters. The struggle between elegance and clumsiness is ongoing and shows no signs of a final victorious resolution.

Now to String Theory—is it beautiful, as string theorists will tell you, or is it the overcomplicated monstrosity that critics of the theory claim? But before discussing the esthetics, let me fill you in about why we need String Theory at all. If, as I said, the Standard Model describes all known phenomena, why are theoretical physicists driven to search for a deeper mathematical structure? The answer is that the Standard Model does not describe all known phenomena. There is at least one obvious exception: gravity. Gravitation is the most familiar force in daily life, and probably the most fundamental, but it is not found anywhere in the Standard Model. The graviton (the quantum of the gravitational field) is not on the list of Standard Model particles. Perhaps the most interesting objects of all, black holes, have no place in the theory. Although Einstein’s classical theory of gravity may be the most beautiful of all theories, it just doesn’t seem to fit into the quantum world.

For most purposes gravity is completely unimportant for elementary-particle physics. As we will see in later chapters, the gravitational force between particles—for example, quarks in a proton—is many orders of magnitude weaker than the other forces of nature. Gravity is far too feeble to play a role in any experiment involving elementary particles, at least for the foreseeable future. For this reason traditional elementary-particle physicists have been content to completely ignore the effects of gravity.

But there are two practical reasons for wanting a deeper understanding of the connections between gravity and the microscopic quantum world. The first has to do with the structure of elementary particles. Although the gravitational force is negligible for electrons in an atom (or quarks in a proton), as the distance between particles gets smaller, gravity begins to assert itself. All forces become stronger as the separation decreases, but the gravitational force increases more rapidly than any other. In fact by the time two particles get within a Planck distance of each other, the force of gravity is a good deal stronger than electric forces or even the forces that bind quarks together. If the “Russian nesting doll” paradigm (things made of ever smaller things) continues to hold sway, the ordinary elementary particles may turn out to be made of even tinier objects that are in some sense held together by gravity.

The second practical reason for understanding the links between gravity and quantum theory involves cosmology. In the next chapter we will see that gravity is the force that governs the growth of the universe. When the universe was very young and expanding at a stupendous rate, gravity and quantum mechanics were important in equal measure. A lack of understanding of the connection between these two great theories will ultimately frustrate our efforts to get to the bottom of the Big Bang.

But there is a third reason that physicists are driven to combine quantum theory with general relativity: an esthetic reason. For a physicist, unlike a poet, the biggest crime against esthetics is the crime of inconsistency. Even worse than an ugly theory, incompatible principles are an attack on the basic values we hold dear. And for most of the twentieth century, gravity and quantum mechanics have been incompatible.

That’s where String Theory comes in. We won’t get to the details of String Theory until chapter 7, but for now let us just say that it is a mathematical theory that consistently unifies gravity with quantum mechanics. Many theoretical physicists (I count myself among them) have a strong feeling that String Theory is our best hope for eventually reconciling these two great but clashing pillars of modern science. What is it about String Theory that makes us feel that way? We have tried lots of other approaches, but they typically flop at the outset. An example is the attempt to build a quantum field theory based on general relativity. The mathematics rapidly becomes inconsistent. But even if the equations made sense, there would be an esthetic disappointment. In every such attempt gravity is an optional “add-on.” By that I mean that gravity is just added to some preexisting theory such as Quantum Electrodynamics. There is nothing at all inevitable about it. But String Theory is different. The existence of both gravity and quantum mechanics is absolutely essential for mathematical consistency. String Theory makes sense
only
as a theory of “quantum gravity.” That is not a small thing, given the way these two giants—gravity and quantum mechanics—have been at war with each other for most of the twentieth century. I would say that this inevitability is beautiful.

In addition to its close connection with gravity, String Theory looks as if it has connections with ordinary elementary-particle physics. By no means have we understood exactly how to incorporate the Standard Model into String Theory, but it does have all the elements that go into modern particle theory. It has particles—fermions and bosons—that resemble electrons, quarks, photons, gluons, and all of the others. In addition to gravitational forces, at work are forces similar to electric and magnetic forces between charged particles and even forces similar to those that bind quarks into protons and neutrons. None of these things is put in by hand. Like gravity, they also are inevitable mathematical consequences of the theory.