Warped Passages (58 page)

The same argument applies to rolled-up dimensions in a universe with any number of curled-up dimensions. The larger the volume of the extra dimensions, the more dilute the gravitational force and the weaker the strength of gravity. We can see this with a higher-dimensional hose analogous to the one we just considered. Gravitational force lines in a higher-dimensional hose would first spread out in all dimensions, including the extra curled-up dimensions. The force lines would reach the boundary of the curled-up dimensions, after which they would spread out only along the infinite dimensions of the lower-dimensional space. The initial spreading out in the extra dimensions would reduce the density of force lines in the lower-dimensional space, so the strength of gravity experienced there would be weaker.
34
Back to the Hierarchy Problem
Because of the dilution of gravity in extra dimensions, lower-dimensional gravity is weaker when the volume of the extra-dimensional compactified space is bigger. ADD observed that this dilution of gravity into extra dimensions could conceivably be so large that it could account for the observed weakness of four-dimensional gravity in our world.
They reasoned as follows. Suppose that gravity in a higher-dimensional theory does not depend on the enormous Planck scale
mass of 10
19
GeV, but instead on a much smaller energy, about a TeV, sixteen orders of magnitude smaller. They chose a TeV to eliminate the hierarchy problem: if a TeV or some nearby energy were the energy at which gravity became strong, there would be no hierarchy of masses in particle physics. Everything, both particle physics and gravity, would be characterized by the TeV scale. So maintaining a reasonably light Higgs particle with mass of about a TeV would not be a problem in their model.
According to their assumption, at energies of about a TeV higher-dimensional gravity would be a reasonably strong force, comparable in strength to the other known forces. To have a sensible theory that agrees with what we see, ADD therefore needed to explain why four-dimensional gravity looks so weak. The added ingredient in their model was the assumption that the extra dimensions are extremely large. Ultimately we would want to explain this large size. But according to their proposal, the curled-up dimensions enclose such a large volume. And, in keeping with the logic of the previous section, four-dimensional gravity would be extremely feeble. Gravity in our world would be weak because extra dimensions are large, not because there is fundamentally a big mass responsible for the tiny gravitational force. The Planck scale mass that we measure in four dimensions is large (making gravity appear weak) only because gravity has been diluted in large extra dimensions.
How large would these extra dimensions have to be? The answer depends on the number of extra dimensions. ADD considered different possible numbers of dimensions for their model, since experiments haven’t yet decided how many dimensions there are. Notice that we are interested only in the large dimensions at this point. So if you think that you and your local string theorist know that the number of spatial dimensions is nine or ten, you can still consider different possibilities for the number of large dimensions and assume that all the other dimensions are small enough to ignore.
The size of the dimensions in the ADD proposal depends on how many there are, because volume depends on the number of dimensions. If all dimensions were the same size, a higher-dimensional region would enclose more volume than a lower-dimensional one and would therefore dilute gravity more. You can see this easily enough
from the fact that lower-dimensional objects fit inside higher-dimensional ones. Or, returning to our sprinkler analogy in Chapter 2, you can see that a plant receives more water from a sprinkler that spreads water only over a line segment of a particular size (one dimension) than one that spreads water over the surface area enclosed by a circle (two dimensions) whose diameter is the same size. When water spreads over a higher-dimensional region, it becomes more diluted.
If there were only one large extra dimension, it would have to be enormous to satisfy the ADD proposal. It would have to be as large as the distance from the Earth to the Sun in order to dilute gravity enough. That’s not allowed. If the extra dimension were that big, the universe would behave as if it were five-dimensional at measurable distances. We already know that Newton’s gravitational force law applies at these distances; a large extra dimension that would modify gravity at such large distances is clearly ruled out.
However, with as few as two additional dimensions, the size of the dimensions is almost acceptably small. If there were just two additional dimensions, they could be as small as a millimeter and still adequately dilute gravity. That is the reason ADD paid so much attention to the millimeter scale. Not only was it on the verge of experimental probes, but two additional dimensions of this size could be relevant to the hierarchy problem. Gravity would spread throughout these two millimeter-size dimensions and yield the weak gravitational force we know. Of course, a millimeter is still pretty big, but as we said earlier, gravity tests are not nearly as restrictive as you might think. Spurred on by the ADD scenario, people thought harder about looking for rolled-up dimensions of this size.
With more than two additional dimensions, gravity is modified only at a very small distance. With more additional dimensions, it can be sufficiently diluted even if those extra dimensions are relatively small. For example, with six extra dimensions the size need only be about 10
-13
cm, one ten thousandth of a billionth of a centimeter.
Even with such small dimensions we could, if we’re lucky, find evidence of one of these examples some time very soon—not in the direct gravitational tests we’ll discuss in the next section, but in the experiments at high-energy particle colliders that we’ll consider afterwards.
Looking for Large Dimensions
How would one go about finding differences in gravity at small distances? What should one look for? We know that if there are curled-up dimensions, the strength of gravity at distances less than the size of the extra dimensions would decrease more rapidly with distance than Newton had predicted, because gravity would spread out in more than three spatial dimensions. Whenever objects were separated by less than the extra dimensions’ sizes, higher-dimensional gravity would apply. A bug sufficiently small to circle a curled-up dimension would experience the extra dimension, both because it could travel in it and because the gravitational force would spread around it in all dimensions. So if anyone, such as this unusually perceptive bug, could detect the gravitational force at short distances, extra dimensions would have visible consequences.
This tells us that by exploring gravity at distances as small as (or smaller than) a proposed curled-up dimension’s size, and studying how gravity’s strength depends on the separation of masses at those distances, an experiment could study the behavior of gravity and look for evidence of extra dimensions. However, experiments that are sensitive to gravity at very short distances are formidably difficult to build. Gravity is so weak that it is readily overwhelmed by the other forces, such as electromagnetism. As mentioned earlier, at the time of the ADD proposal, experiments had searched for deviations from Newton’s gravitational force law and shown that the law applies at least down to distances of about a millimeter. If anyone could do better and study even shorter distances, they had a chance of discovering the large dimensions of the ADD proposal, which were just on the verge of experimental accessibility.
Experimenters rose to the new challenge. Motivated by the ADD idea, Eric Adelberger and Blayne Heckel, two professors at the University of Washington, designed a beautiful experiment whose purpose was to look for deviations from Newton’s law at very short distances. Others have also studied short-distance gravity, but this experiment was the most stringent test of the ADD proposal.
Their apparatus, located in the basement of the University of
Washington physics department, is called the Eöt-Wash experiment. The name refers to a famous physicist who studied gravity, the Hungarian Baron Roland von Eötvös. The Eöt-Wash group’s experiment is illustrated in Figure 76. It consists of a ring suspended above two attractor disks, one slightly above the other. Holes are bored into the ring, and the upper and lower disks, and they are aligned in just such a way that if Newton’s law is correct, the ring won’t twist. However, if there were extra dimensions, the difference in gravitational attraction from the two disks would not agree with Newton’s law and the ring would twist.
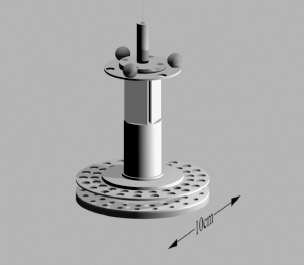
Figure 76.
The apparatus of the Eöt-Wash experiment. A ring is suspended over two disks. The holes in the ring and the disks guarantee that the ring will not twist if Newton’s inverse square law is obeyed. The three spheres near the top of the apparatus are used for calibration purposes.
Adelberger and Heckel found no twisting and concluded that no extra-dimensional (or other) effects modified the gravitational force at the distances they could study. Their experiment measured the gravitational force at distances smaller than ever before, establishing that Newton’s law applies all the way down to about a tenth of a millimeter. This meant that extra dimensions, even those for which Standard Model particles are confined on a brane, cannot be quite as
big as the millimeter that ADD had suggested. They have to be at least ten times smaller.
Remarkably, millimeter-size dimensions are also prohibited by observations of outer space. The quantum mechanical uncertainty principle associates a millimeter with an energy of only about 10
-3
eV, and a tenth of a millimeter with an energy of about 10
-2
eV—either way, an extremely small energy, orders of magnitude less than that needed to produce an electron, for example.
Particles with such a low mass could be found in the surrounding universe and in celestial objects, such as supernovae or the Sun. These particles would be so light that if they existed, hot supernovae could produce them. Because we know how quickly supernovae cool and we understand the cooling mechanism (via neutrino emission), we know that there can’t be too many other low-mass objects emitted. The cooling rate would be too fast if energy leaked out in some other way. In particular, gravitons shouldn’t carry away too much energy. Using this reasoning, physicists showed (independently of terrestrial experiments) that extra dimensions should be smaller than about a hundredth of a millimeter.
However, you should bear in mind that, impressive as it is to rule out deviations of gravity at millimeter distances, this doesn’t test most of the currently proposed extra-dimensional models. Remember, only the model with two large extra dimensions produces effects that would be visible on the millimeter scale. If a theory with more than two large extra dimensions solves the hierarchy problem (or if one of the models we’ll consider in the next chapter applies to the world), deviations from Newton’s law would occur only at much shorter distances.
We don’t know for sure what the gravitational attraction between two objects less than a tenth of a millimeter apart will look like. No one has ever tested it. So we don’t know whether extra dimensions open up at a tenth of a millimeter, which, if you think about it, is not all that small. Relatively large extra dimensions—though not quite as big as a millimeter—remain a viable possibility. To test such models we’ll have to wait for collider tests, the subject of the next section.
Collider Searches for Large Extra Dimensions
High-energy particle colliders are well-suited to discover KK particles from large extra dimensions, even if there are more than two of them. In the ADD large extra-dimensions models, the KK partners of the graviton are always incredibly light. If the large-dimension proposal applies to the real world, the graviton KK partners would be light enough to be produced at accelerators, no matter how many extra dimensions there were. That tells us that even if dimensions are smaller than a millimeter, current and future accelerator searches should be able to discover them. Current colliders create more than enough energy to make such low-mass particles. In fact, if the only relevant quantity were energy, KK particles would already have been produced in abundance.
However, there is a catch. The graviton’s KK partners interact only incredibly feebly—as feebly, in fact, as the graviton itself. Since a graviton’s interactions are so negligible that gravitons are never produced or detected at colliders at a measurable rate, an individual graviton KK partner wouldn’t be either.
But the potential for detecting KK particles from higher dimensions is actually much more promising than this dismal assessment might lead you to believe. This is because, if the ADD proposal is correct, there would be so many light KK partners of the graviton that together they could leave detectable evidence of their existence. If the large-dimensions scenario is true, then even though any individual KK particle could be produced only rarely, the probability of producing one of the large number of light KK particles would be measurably large. For example, if there were two extra dimensions, about one hundred billion trillion KK modes would be light enough to be produced at a collider operating at an energy of about a TeV. The rate of producing at least one of these particles would be fairly high, even if the rate of producing any single one of them were extremely low.
