Broca's Brain (23 page)
Authors: Carl Sagan

Bloom has issued a fascinating pamphlet, which states: “The complete faculty of Princeton University (including its officers and its deans and the chairmen of the departments listed here) has agreed that it cannot refute, nor show in basic error the proof brought to it, in the book,
The New World
dated Sept. 1974. This faculty acknowledges as of June 1, 1975 that it accepts as a proven truth THE IRREFUTABLE PROOF THAT AN ETERNAL MIND AND HAND HAS SHAPED AND CONTROLLED THE HISTORY OF THE WORLD THROUGH THOUSANDS OF YEARS.” A closer reading shows that despite Bloom’s distributing his proofs to over a thousand faculty members of Princeton University, and despite his offer of a $1,000 prize for the first individual to refute his proof, there was no response whatever. After six months he concluded that since Princeton did not answer, Princeton believed. Considering the ways of university faculty members, an alternative explanation has occurred to me. In any case, I do not think that the absence of a reply constitutes irrefutable support for Bloom’s arguments.
Princeton has apparently not been alone in treating Bloom inhospitably: “Yes, times almost without number, I have been chased by police for bringing you the gift of my writing … Is it not so that professors at a university are supposed to have the maturity and judgment
and wisdom to be able to read a writing and determine for themselves the value of its contents? Is it that they require THOUGHT CONTROL POLICE to tell them what they should or should not read or think about? But, even at the astronomy department of Harvard University, I have been chased by police for the crime of distributing that New World Lecture, an irrefutable proof that the earth-moon-sun system is shaped by a controlling mind and hand. Yes, and THREATENED WITH IMPRISONMENT, IF I DARE BESMIRCH THE HARVARD CAMPUS WITH MY PRESENCE ONCE MORE.… AND THIS IS THE UNIVERSITY THAT HAS UPON ITS SHIELD THE WORD VERITAS: VERITAS: VERITAS:—Truth, Truth, Truth. Ah, what hypocrites and mockers you are!”
The supposed proofs are many and diverse, all involving numerical coincidences which Bloom believes could not be due to chance. Both in style and content, the arguments are reminiscent of Talmudic textual commentary and cabalistic lore of the Jewish Middle Ages: for example, the angular size of the Moon or the Sun as seen from the Earth is half a degree. This is just 1/720 of the circle (360°) of the sky. But 720 = 6! = 6 × 5 × 4 × 3 × 2 × 1. Therefore, God exists. It is an improvement on Euler’s proof to Diderot, but the approach is familiar and infiltrates the entire history of religion. In 1658 Gaspar Schott, a Jesuit priest, announced in his
Magia Universalis Naturae et Artis
that the number of degrees of grace of the Virgin Mary is 2
256
= 2
2
8 1.2 × 10
1.2 × 10
77
(which, by and by, is very roughly the number of elementary particles in the universe).
Another Bloomian argument is described as “irrefutable proof that the God of Scripture is he who has shaped and controlled the history of the world through thousands of years.” The argument is this: according to Chapters 5 and 11 of Genesis, Abraham was born 1,948 years after Adam, at a time when Abraham’s father, Terah, was seventy years old. But the Second Temple was destroyed by the Romans in
A.D.
70, and
the State of Israel was created in
A.D.
1948 Q.E.D. It is hard to escape the impression that there may be a flaw in the argument somewhere. “Irrefutable” is, after all, a fairly strong word. But the argument is a refreshing diversion from St. Anselm.
Bloom’s central argument, however, and the one that much of the rest is based upon, is the claimed astronomical coincidence that 235 new moons is, with spectacular accuracy, just as long as nineteen years. Whence: “Look, mankind, I say to you all, in essence you are living in a clock. The clock keeps perfect time, to an accuracy of one second/day!… How could such a clock in the heavens come to be without there being some being, who with perception and understanding, who, with a plan and with the power, could form that clock?”
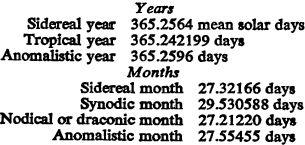
A fair question. To pursue it we must realize that there are several different kinds of years and several different kinds of months in use in astronomy. The sidereal year is the period that the Earth takes to go once around the Sun with respect to the distant stars. It equals 365.2564 days. (The days we will use, as Norman Bloom does, are what astronomers call “mean solar days.”) Then there is the tropical year. It is the period for the Earth to make one circuit about the Sun with respect to the seasons, and equals 365.242199 days. The tropical year is different from the sidereal year because of the precession of the equinoxes, the slow toplike movement of the Earth produced by the gravitational forces of the Sun and the Moon on its oblate shape. Finally, there is the so-called anomalistic year of 365.2596 days. It is the interval between two successive closest approaches of the Earth to the Sun, and is different from the sidereal year because of the slow movement of the Earth’s elliptical orbit in its own plane, produced by gravitational tugs by the nearby planets.
Likewise, there are several different kinds of months. The word “month,” of course, comes from “moon.” The sidereal month is the time for the Moon to go once around the earth with respect to the distant stars and
equals 27.32166 days. The synodic month, also called a lunation, is the time from new moon to new moon or full moon to full moon. It is 29.530588 days. The synodic month is different from the sidereal month because, in the course of one sidereal revolution of the Moon about the Earth, the Earth-Moon system has together revolved a little bit (about one-thirteenth) of the way around the Sun. Therefore the angle by which the Sun illuminates the Moon has changed from our terrestrial vantage point. Now, the plane of the Moon’s orbit around the Earth intersects the plane of the Earth’s orbit around the Sun at two places—opposite to each other—called the nodes of the Moon’s orbit. A nodical or draconic month is the time for the Moon to move from one node back around again to the same node and equals 27.21220 days. These nodes move, completing one apparent circuit, in 18.6 years because of gravitational tugs, chiefly by the Sun. Finally, there is the anomalistic month of 27.55455 days, which is the time for the Moon to complete one circuit of the Earth with respect to the nearest point in its orbit. A little table on these various definitions of the year and the month is shown below.
KINDS OF YEARS AND MONTHS,
EARTH-MOON SYSTEM
Now, Bloom’s main proof of the existence of God depends upon choosing one of the sorts of years, multiplying it by 19 and then dividing by one of the sorts of months. Since the sidereal, tropical and anomalistic years are so close together in length, we get sensibly the same answer whichever one we choose. But the same is
not true for the months. There are four different kinds of months, and each gives a different answer. If we ask how many
synodic
months there are in nineteen sidereal years, we find the answer to be 253.00621, as advertised; and it is the closeness of this result to a whole number that is the fundamental coincidence of Bloom’s thesis. Bloom, of course, believes it to be no coincidence.
But if we were to ask instead how many
sidereal
months there are in nineteen sidereal years we would find the answer to be 254.00622; for nodical months, 255.02795; and for anomalistic months, 251.85937. It is certainly true that the synodic month is the one most strikingly apparent to a naked-eye observer, but I nevertheless have the impression that one could construct equally elaborate theological speculations on 252, 254, or 255 as on 235.
We must now ask where the number 19 comes from in this argument. Its only justification is David’s lovely Nineteenth Psalm, which begins: “The heavens declare the glory of God, and the firmament sheweth his handiwork. Day unto day uttereth speech, and night unto night sheweth knowledge.” This seems quite an appropriate quotation from which to find a hint of an astronomical proof for the existence of God. But the argument assumes what it intends to prove. The argument is also not unique. Consider, for example, the Eleventh Psalm, also written by David. In it we find the following words, which may equally well bear on this question: “The Lord is in his holy temple, the Lord’s throne is in heaven: his eyes behold, his eyelids try, the children of men,” which is followed in the following Psalm with “the children of men … speak vanity.” Now, if we ask how many synodic months there are in eleven sidereal years (or 4017.8204 mean solar days), we find the answer to be 136.05623. Thus, just as there seems to be a connection between nineteen years and 235 new moons, there is a connection between eleven years and 136 new moons. Moreover, the famous British astronomer Sir Arthur Stanley Eddington believed that all of physics could be derived from the number 136.
(I once suggested to Bloom that with the foregoing information and just a little intellectual fortitude it should be possible as well to reconstruct all of Bosnian history.)
One numerical coincidence of this sort, which
is
of deep significance, was well known to the Babylonians, contemporaries of the ancient Hebrews. It is called the Saros. It is the period between two successive similar cycles of eclipses. In a solar eclipse the Moon, which appears from the Earth just as large (1/2°) as the Sun, must pass in front of it. For a lunar eclipse, the Earth’s shadow in space must intercept the Moon. For either kind of eclipse to occur, the Moon must, first of all, be either new or full—so that the Earth, the Moon and the Sun are in a straight line. Therefore the synodic month is obviously involved in the periodicity of eclipses. But for an eclipse to occur, the Moon must also be near one of the nodes of its orbit. Therefore the nodical month is involved. It turns out that 233 synodic months is equal to 241.9989 (or very close to 242) nodical months. This is the equivalent of a little over eighteen years and ten or eleven days (depending on the number of intervening leap days), and comprises the Saros. Coincidence?
Similar numerical coincidences are in fact common throughout the solar system. The ratio of spin period to orbital period on Mercury is 3 to 2. Venus manages to turn the same face to the Earth at its closest approach on each of its revolutions around the Sun. A particle in the gap between the two principal rings of Saturn, called the Cassini Division, would orbit Saturn in a period just half that of Mimas, its second satellite. Likewise, in the asteroid belt there are empty regions, known as the Kirkwood Gaps, which correspond to nonexistent asteroids with periods half that of Jupiter, one-third, two-fifths, three-fifths, and so on.
None of these numerical coincidences proves the existence of God—or if it does, the argument is subtle, because these effects are due to resonances. For example, an asteroid that strays into one of the Kirkwood Gaps experiences a periodic gravitational pumping by
Jupiter. Every two times around the Sun for the asteroid, Jupiter makes exactly one circuit. There it is, tugging away at the same point in the asteroid’s orbit every revolution. Soon the asteroid is persuaded to vacate the gap. Such incommensurable ratios of whole numbers are a general consequence of gravitational resonance in the solar system. It is a kind of perturbational natural selection. Given enough time—and time is what the solar system has a great deal of—such resonances will arise inevitably.
That the general result of planetary perturbations is stable resonances and not catastrophic collisions was first shown from Newtonian gravitational theory by Pierre Simon, Marquis de Laplace, who described the solar system as “a great pendulum of eternity, which beats ages as a pendulum beats seconds.” Now, the elegance and simplicity of Newtonian gravitation might be used as an argument for the existence of God. We could imagine universes with other gravitational laws and much more chaotic planetary interactions. But in many of those universes we would not have evolved—precisely because of the chaos. Such gravitational resonances do not prove the existence of God, but if he does exist, they show, in the words of Einstein, that, while he may be subtle, he is not malicious.