Gödel, Escher, Bach: An Eternal Golden Braid (54 page)
Read Gödel, Escher, Bach: An Eternal Golden Braid Online
Authors: Douglas R. Hofstadter
Tags: #Computers, #Art, #Classical, #Symmetry, #Bach; Johann Sebastian, #Individual Artists, #Science, #Science & Technology, #Philosophy, #General, #Metamathematics, #Intelligence (AI) & Semantics, #G'odel; Kurt, #Music, #Logic, #Biography & Autobiography, #Mathematics, #Genres & Styles, #Artificial Intelligence, #Escher; M. C

clearest Zen statements about the borderlines of Zen is given in the fol ing strange koan, very much in the spirit of Nansen:10
Tozan said to his monks, "You monks should know there is an even high understanding in Buddhism." A monk stepped forward and asked, "What the higher Buddhism?" Tozan answered, "It is not Buddha."
There is always further to go; enlightenment is not the end-all of And there is no recipe which tells how to transcend Zen; the only thing can rely on for sure is that Buddha is not the way. Zen is a system cannot be its own metasystem; there is always something outside of which cannot be fully understood or described within Zen.
Escher and Zen
In questioning perception and posing absurd answerless riddles, Zen company, in the person of M. C. Escher. Consider Day and Night (Fig. 4 masterpiece of "positive and negative interwoven" (in the words of Mumoni). One might ask, "Are those really birds, or are they really field it really night, or day?" Yet we all know there is no point to such questions The picture, like a Zen koan, is trying to break the mind of logic. Es4 also delights in setting up contradictory pictures, such as
Another World
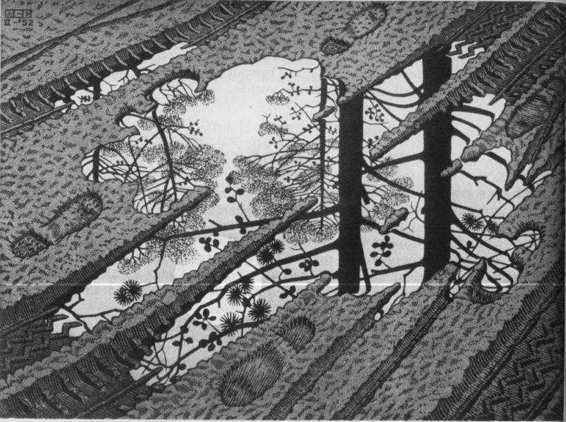
FIGURE 51. Puddle, by M. C. Escher (woodcut, 1952).
(Fig. 48)-pictures that play with reality and unreality the same way as Zen plays with reality and unreality. Should one take Escher seriously? Should one take Zen seriously?
There is a delicate, haiku-like study of reflections in
Dewdrop
(Fig. 47); and then there are two tranquil images of the moon reflected in still waters:
Puddle
(Fig. 51), and
Rippled Surface
(Fig. 52). The reflected moon is a theme which recurs in various koans.
Here is an example:'
Chiyono studied Zen for many years under Bukko of Engaku. Still, she could not attain the fruits of meditation. At last one moonlit night she was carrying water in an old wooden pail girded with bamboo. The bamboo broke, and the bottom fell out of the pail. At that moment, she was set free. Chiyono said, "No more water in the pail, no more moon in the water."
Three Worlds
: an Escher picture (Fig. 46), and the subject of a Zen koan:12
A monk asked Ganto, "When the three worlds threaten me, what shall I do?" Ganto answered, "Sit down." "I do not understand," said the monk. Canto said, "Pick up the mountain and bring it to me. Then I will tell you."

Hemiolia and Escher
In Verbum (Fig. 149), oppositions are made into unities on several I Going around we see gradual transitions from black birds to white birds to black fish to white fish to black frogs to white frogs to black birds ... six steps, back where we started! Is this a reconciliation of the dichotomy of black and white? Or of the trichotomy of birds, fish, and frogs? Or sixfold unity made from the opposition of the evenness of 2 an oddness of 3? In music, six notes of equal time value create a rhythmic ambiguity-are they 2 groups of 3, or 3 groups of 2? This ambiguity has a name: hemiolia. Chopin was a master of hemiolia: see his Waltz op. his Etude op. 25, no. 2. In Bach, there is the Tempo di Menuetto from the keyboard Partita no. 5, or the incredible Finale of the first Sonata unaccompanied violin, in G Minor.
As one glides inward toward the center of Verbum, the distinctions gradually blur, so that in the end there remains not three, not two, but one single essence: "VERBUM", which glows with brilliancy-perhaps a symbol of enlightenment. Ironically, ` verbum"
not only is a word, but "word"-not exactly the most compatible notion with Zen. On the hand, "verbum" is the only word in the picture. And Zen master 1 once said, "The complete Tripitaka can be expressed in one character ("Tripitaka", meaning "three baskets", refers to the complete texts c original Buddhist writings.) What kind of decoding-mechanism, I wonder would it take to suck the three baskets out of one character? Perhaps one with two hemispheres.
FIGURE 52. Rippled Surface, by M. C. Escher (lino-cut, 1950).
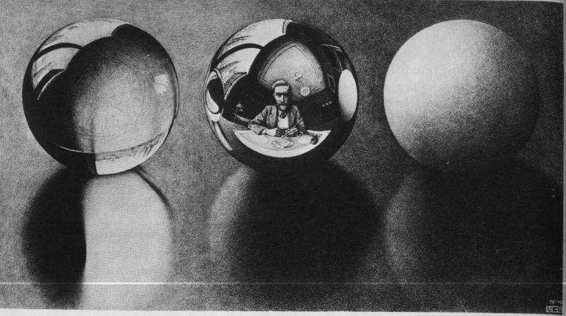
FIGURE 53. Three Spheres II, by M. C. Escher (lithograph, 1946),
Indra's Net
Finally, consider
Three Spheres II
(Fig. 53), in which every part of the world seems to contain, and be contained in, every other part: the writing table reflects the spheres on top of it, the spheres reflect each other, as well as the writing table, the drawing of them, and the artist drawing it. The endless connections which all things have to each other is only hinted at here, yet the hint is enough. The Buddhist allegory of "Indra's Net" tells of an endless net of threads throughout the universe, the horizontal threads running through space, the vertical ones through time. At every crossing of threads is an individual, and every individual is a crystal bead. The great light of "Absolute Being" illuminates and penetrates every crystal bead; moreover, every crystal bead reflects not only the light from every other crystal in the net-but also every reflection of every reflection throughout the universe.
To my mind, this brings forth an image of renormalized particles: in every electron, there are virtual photons, positrons, neutrinos, muons ... ; in every photon, there are virtual electrons, protons, neutrons, pions ... ; in every pion, there are ...
But then another image rises: that of people, each one reflected in the minds of many others, who in turn are mirrored in yet others, and so on.
Both of these images could be represented in a concise, elegant way by using Augmented Transition Networks. In the case of particles, there would be one network for each category of particle; in the case of people,
one for each person. Each one would contain calls to many others, t creating a virtual cloud of ATN's around each ATN. Calling one we create calls on others, and this process might cascade arbitrarily far, un~ bottomed out.
Mumon on MU
Let us conclude this brief excursion into Zen by returning to Mumon. H is his comment on Joshu's MU:13
To realize Zen one has to pass through the barrier of the patriarchs. Enlightenment always comes after the road of thinking is blocked. If you do nc pass the barrier of the patriarchs or if your thinking road is not blocked whatever you think, whatever you do, is like a tangling ghost. You may ask "What is a barrier of a patriarch?" This one word, 'MU', is it.
This is the barrier of Zen. If you pass through it, you will see Joshu face t face.
Then you can work hand in hand with the whole line of patriarchs. I this not a pleasant thing to do?
If you want to pass this barrier, you must work through every bone in you body, through every pore of your skin, filled with this question: "What `MU'?" and carry it day and night. Do not believe it is the common negative symbol meaning nothing. It is not nothingness, the opposite of existence. I you really want to pass this barrier, you should feel like drinking a hot iro ball that you can neither swallow nor spit out.
Then your previous lesser knowledge disappears. As a fruit ripening i season, your subjectivity and objectivity naturally become one. It is like dumb man who has had a dream. He knows about it but he cannot tell i
When he enters this condition his ego-shell is crushed and he can shake th heaven and move the earth. He is like a great warrior with a sharp sword. If Buddha stands in his way, he will cut him down; if a patriarch offers him an obstacle, he will kill him; and he will be free in his way of birth and death. H can enter any world as if it were his own playground. I will tell you how to d this with this koan: Just concentrate your whole energy into this MU, and do not allow an discontinuation. When you enter this MU and there is no discontinuation -- your attainment will be as a candle burning and illuminating the who] universe.
From Mumon to the MU-puzzle
From the ethereal heights of Joshu's MU, we now descend to the private lowlinesses of Hofstadter's MU . . . I know that you have already concentrated your whole energy into this MU (when you read Chapter 1). So n wish to answer the question which was posed there:
Has
MU
theorem-nature, or not?
The answer to this question is not an evasive MU; rather, it is a resounding NO. In order to show this, we will take advantage of dualistic, logical thinking.
We made two crucial observations in Chapter I:
(1) that the MU-puzzle has depth largely because it involves the interplay of lengthening and shortening rules;
(2) that hope nevertheless exists for cracking the problem by employing a tool which is in some sense of adequate depth to handle matters of that complexity: the theory of numbers.
We did not analyze the MU-puzzle in those terms very carefully in Chapter I; we shall do so now. And we will see how the second observation (when generalized beyond the insignificant MIU-system) is one of the most fruitful realizations of all mathematics, and how it changed mathematicians' view of their own discipline.
For your ease of reference, here is a recapitulation of the MIU-system: SYMBOLS: M, I, U
Axiom: MI
RULES:
I. If xl is a theorem, so is xIU.
II. If Mx is a theorem, so is Mxx.
III. In any theorem, III can be replaced by U.
IV. UU can be dropped from any theorem.
Mumon Shows Us How to Solve the MU-puzzle
According to the observations above, then, the MU-puzzle is merely a puzzle about natural numbers in typographical disguise. If we could only find a way to transfer it to the domain of number theory, we might be able to solve it. Let us ponder the words of Mumon, who said, "If any of you has one eye, he will see the failure on the teacher's part." But why should it matter to have one eye?
If you try counting the number of l's contained in theorems, you will soon notice that it seems never to be 0. In other words, it seems that no matter how much lengthening and shortening is involved, we can never work in such a way that all I's are eliminated.
Let us call the number of I's in any string the I-count of that string. Note that the I-count of the axiom MI is 1. We can do more than show that the I-count can't be 0-we can show that the I-count can never be any multiple of 3.
To begin with, notice that rules I and IV leave the I-count totally undisturbed.
Therefore we need only think about rules II and III. As far as rule III is concerned, it diminishes the I-count by exactly 3. After an application of this rule, the I-count of the output might conceivably be a multiple of 3-but only if the I-count of the input was also.
Rule III, in short, never creates a multiple of 3 from scratch. It can only create one when it began with one. The same holds for rule II, which doubles the
