Secrets of Antigravity Propulsion (2 page)

During the first few seconds that the voltage was applied, the slowly responding dielectric, for the most part, would have remained unpolarized.
Hence the applied electric field, along with its associated gravitic field effect, would have extended with full intensity throughout the gravitator, exerting a maximal gravitic thrust on the dielectric in the direction of the gravitator’s positive pole.
However, as the dielectric became increasingly polarized, its oppositely directed electric dipole moment field arising within the dielectric would have progressively increased in strength, progressively canceling out the gravitic effects induced by the externally applied electric field.
Thus the thrust pushing the gravitator in the direction of its positive pole would have progressively subsided.
Moreover, when the dielectric reached its fully polarized state with its opposed dipole moment field at its maximum, this thrust would have become almost entirely canceled out, leaving the gravitator to return to its plumb position.
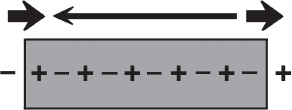
Figure 1.5.
The polarized charge arrangement in the gravitator’s dielectric when voltage is applied to the gravitator plates.
Arrows indicate the direction of the electrogravitic force.
As the dielectric became progressively polarized, the gravitator capacitor plates would have been able to hold an increasing amount of electric charge as an increasing number of polarized molecular charges moved adjacent to the plates to attract additional charges.
As a result, throughout this polarization interval the gravitator would have been charging up and a current would have been flowing to its plates.
Charge would have been accumulating most rapidly in the beginning and the charging rate would have progressively dropped off as the full charged state was approached.
Similarly, the reverse gravitic thrust generated by the polarizing dielectric would have caused the overall gravitic thrust to decline most rapidly at the beginning of the pendulum’s swing and to subside more slowly as the fully charged state was approached.
The observation that the gravitic force subsided in steps may be an indication that the dielectric experienced a succession of abrupt mechanical shifts in its approach to the fully polarized state.
The need to recycle the gravitator between test runs, to discharge it and let it rest so as to “regain its former gravitic condition,” is understandable if we realize that it was necessary to allow a sufficiently long rest period for the dielectric to completely depolarize.
After the DC voltage supply is shut off, a residual charge will initially remain on the capacitor plates, kept there by the dielectric’s residual polarization.
Engineers refer to this remnant charge as
dielectric absorption
.
It is particularly important in capacitors that are capable of storing a lot of charge.
As the dielectric progressively relaxes, this charge is gradually released.
Once the gravitator dielectric had relaxed to an unpolarized state, new charges would be able to rapidly accumulate on its electrodes during the next charging cycle.
Once again, a steep gravity potential gradient would have been able to form across the gravitator and temporarily exert a net thrust on its massive dielectric until it was again opposed by the dielectric’s progressively increasing dipole moment field.
1.3 • A THEORY OF ELECTROGRAVITICS
In August 1927, Brown filed for a British patent on his gravitator idea, which was issued to him in November 1928 (British patent 300,311).
In the patent’s text, Brown clearly proclaims that the propelling force he has discovered is of an unconventional nature:
The invention also relates to machines or apparatus requiring electrical energy that control or influence the gravitational field or the energy of gravitation; also to machines or apparatus requiring electrical energy that exhibit a linear force or motion which is believed to be independent of all frames of reference save that which is at rest relative to the universe taken as a whole, and said linear force or motion is furthermore believed to have no equal and opposite reaction that can be observed by any method commonly known and accepted by the physical science to date.
8
Here he describes his belief that electrogravitic force operates relative to a unique reference frame that is at rest in relation to the universe, an idea that challenges special relativity’s notion that a force should operate in the same manner relative to any frame of reference.
Moreover, he suggests that this force is reactionless when producing its forward thrust—that is, it produces its forward thrust without any back-directed recoil.
He is in effect suggesting that it violates Newton’s third law of motion—that every action should produce an equal and opposite reaction.
Dr.
Patrick Cornille, who repeated Brown’s high-voltage pendulum experiment, came to the similar conclusion that Newton’s third law of motion was indeed violated (see
chapter 12
).
On October 28, 1928, just prior to receiving his patent, Townsend submitted to the physics journal
Physical Review
a paper titled “Tapping Cosmic Energy,” which described his gravitator experiments.
Unfortunately, the journal rejected his paper, apparently because of its unconventional nature.
For one thing, his ideas challenged Einstein’s theory of gravitation, which had by then become staunchly accepted by the physics community.
One year later, Brown published a less technical version of his findings in
Science and Invention Magazine
9
and succeeded in impressing a large number of people with his work.

Figure 1.6.
A gravitator configured within an evacuated envelope reproduced from Brown’s patent.
In this version, the negative electrode or cathode (left) is heated to incandescence, thereby encouraging the thermionic emission of electrons, whereas the positive electrode or anode (right) is cooled by circulating air or water.
This configuration mimics many of the design features of an X-ray tube (or Coolidge tube), like the ones that Brown used when he first observed the electrogravitic phenomenon.
(Brown, 1928)
In 1930 one of Brown’s colleagues wrote about the gravitator to Colonel Edward Deeds, who was one of Brown’s longtime acquaintances.
In his letter he wrote, “I have had a number of scientists view the gravitator and they have all been absolutely amazed at its action, frankly stating that whereas they see the results and the movements of the gravitator, it is absolutely unexplainable by any laws of physics that they know.”
10
At that time, Brown had no theory to explain electrogravity.
It would not be until twenty years later that he sketched out a theory of sorts, which he made notes about in one of his lab notebooks.
But a theoretical methodology that actually predicted charge-mass coupling and that could begin to make some sense out of electrogravitics in a unified-field-theory context did not begin to emerge until the late 1970s with the development of subquantum kinetics.
11,
12,
13
It is useful to review a bit about this theory here, as it will help us interpret the novel results that Brown was getting.
Subquantum kinetics offers an explanation for gravity that is substantially different from Einstein’s relativity theory.
Whereas general relativity postulates that masses exert an attractive gravitational force on other bodies by warping the space-time dimensional fabric around themselves, subquantum kinetics proposes that masses have no such effect on the geometry of space or time.
Subquantum kinetics assumes that space is geometrically flat, or Euclidean; hence, it conforms to the geometrical rules most everyone learns in high school math class.
It predicts that a mass creates a classical gravity potential field and that a gradient in such a field exerts a force on a remote body by affecting how that body’s constituent subatomic particles regenerate their physical form.
(Details of how potential fields are generated and how they accelerate material particles through form regeneration are further discussed in chapter 4.)
Subquantum kinetics also differs from general relativity in its prediction of gravitational field polarity.
According to general relativity, masses only attract other masses, never repel them.
Although Einstein did introduce the notion of a matter-repelling effect whose magnitude he symbolically represented by a quantity called the cosmological constant, this was not part of his general relativity theory, but was an ad hoc correction factor added to his field equations so that they would not predict a universe that was spontaneously contracting due to self-gravitation.
Einstein had attempted to expand his relativity theory to encompass both electromagnetism and gravitation, but he was unsuccessful.
Relativity was unable to predict any connection between charge polarity and gravitational field polarity.
Subquantum kinetics, on the other hand, predicts that gravity should have two polarities.
It permits the creation of either a matter-attracting gravity potential well or a matter-repelling gravity potential hill and predicts that these two gravity polarities should be directly correlated with electric charge polarity.
That is, positively charged particles such as protons would generate gravity wells, whereas negatively charged particles such as electrons would generate gravity hills.
When protons and electrons combine to compose electrically neutral atoms, the gravitational polarities of the protons and electrons for the most part would neutralize one another.
However, because a proton’s gravity well is theorized to marginally exceed an electron’s gravity hill, electrically neutral matter would produce a small, residual matter-attracting gravity potential well, thereby generating the gravity we commonly experience pulling us to Earth.
Subquantum kinetics predicts that a matter-repelling gravity potential hill should form on the negatively charged side of a capacitor and that a matter-attracting gravity potential well forms on the positively charged side.
The intervening gravity potential gradient would produce a gravitational force on the capacitor’s massive dielectric that would act to pull it in the direction of the positively charged plate (figure 1.7).
The more prominent the gravity hill and well, the steeper the gravity potential gradient and the stronger the produced gravitational thrust.
While this force was present, the capacitor would behave as if it was being tugged forward by a very strong gravitational field emanating from an invisible planetary mass situated ahead of its positive pole and as if it was being pushed forward by an equally strong repulsive gravitational force emanating from behind its negative pole.
If the capacitor was placed with its positive pole facing up and was energized such that it generated a sufficiently steep vertical gravity gradient, theoretically the downward pull of gravity could be entirely overcome.
(For a more detailed mathematical analysis of how this electrogravitic force might be quantified, see the
text box
.)
At present there is no easy way to check the prediction that an individual electron might have negative gravitational mass because any matter-repelling gravitational force it might produce would be greatly overpowered by its electrostatic force interactions with surrounding matter.
That is, no one has found a way to screen out these electrostatic forces sufficiently to allow an accurate measurement of a single particle’s gravitational mass.
However, when large numbers of electrons and protons are differentially accumulated, as at the opposite poles of a charged capacitor, the cumulative effect of the negative gravitational potentials of the electrons appears to be great enough to produce an observable macroscopic force.
That force is the electrogravitic effect that Brown observed.
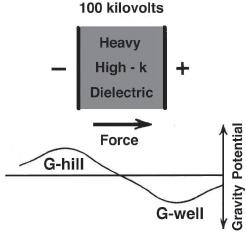
Figure 1.7.
The electro-gravitational force effect produced by charging a capacitor to a high voltage.
(P.
LaViolette, © 1994)
Quantifying the Electrogravitic Effect
Subquantum kinetics, then, predicts that a charged body should generate a gravitational mass, m
g
, that scales directly with the magnitude of its electrical charge.
Their proportional equivalence is expressed by the following electrogravitic coupling relation:
[gravitational mass]
IS PROPORTIONAL TO
[electric charge]
or with symbols:
(1)
m
g
∝
q,
Thus, a body that has a fourfold increase in positive electric charge should produce a fourfold-greater positive gravitational mass.
Also, a fourfold increase in negative electric charge should produce a fourfold-greater negative (mass-repelling) gravitational mass.
Moreover, because electric charge comes in either a positive or negative polarity, ±q, gravitational mass would similarly be induced in either of two polarities correlated with the charge polarity.
The same electrogravitic rule holds when expressed in terms of electric charge
density
, ρ
e
, and gravitational mass
density
, ρ
m
, quantities that refer to the amount of charge or gravitational mass per unit volume.
Their proportional equivalence is expressed as:
[gravitational mass density]
IS PROPORTIONAL TO
[electric charge density]
or with symbols:
(2)
ρ
m
∝
ρ
e
We may also express this charge–mass correspondence in terms of energy potentials or, to use another phrase, in terms of field potentials.
For example, a positively charged body that is characterized by a positive charge density,
ρ
e
, would create a positive electric potential within itself.
This elevated potential would create an electric potential field, φ
e
(
r
), that would appear as an electric potential hill having its maximum centered on the charged body and a magnitude that progressively declined with increasing radial distance
r
from that body.
The parenthetical expression, (r), indicates that the field magnitude varies with distance
r
.
As noted in relation 2, a body having a positive electric charge density would produce a proportionate positive gravitational mass density,
ρ
m
, that would supplement its inherent natural mass density.
This in turn would create a proportional negative gravity potential within the body supplementing its naturally produced negative gravity potential, which in turn would generate an extended gravity potential field –φ
g
(
r
).
This gravity field would be configured as a gravity potential well centered on the charged body, its gravity potential progressively rising to more positive values with increasing radial distance r from that body.
In the case of a negative charge density, these field polarities would be reversed, resulting in an electric potential well centered on the body that in turn would produce a gravity potential hill.
Note that when speaking of gravity fields, what we term a “positive mass” by convention is one that produces a matter-attracting gravity potential well.
In the case of electric charge, on the other hand, by convention a positive charge would produce a positive electric potential hill.
The electrogravitic relations presented in (1) and (2) may be expressed in terms of field potentials as:
[gravity potential]
IS PROPORTIONAL TO
[negative electric potential]
or with symbols:
(3)
φ
g
(
r
)
∝
– φ
e
(
r
).
Hence, an electric potential field gradient extending between the positive and negative plates of a capacitor would produce a proportional gravity potential field gradient of opposite sign across the capacitor’s intervening dielectric; recall figure 1.5.
Also, Newton’s second law tells us that a gravity potential field will generate a force on a body that is proportional to the magnitude of the field gradient multiplied by the body’s inertial mass.
This may be expressed mathematically by the equation:
(4)
F
g(
r
)
=
–Gm
o
∇
φ
g
(
r
),
where
F
g
(
r
) is the gravitational force acting on a body, G is the gravitational constant, mo is the inertial mass of the affected body, and
∇
φ
g
(
r
) is the local gravity potential gradient that is sometimes alternatively symbolized as
grad
φ
g
(
r
).
The bold type on the force and gradient symbols indicates that they are vector quantities having direction as well as magnitude.
Basically this equation states that the steeper the gravity field gradient, the greater the produced force, as was mentioned earlier in connection with figure 1.7.
Or, alternatively, the greater the magnitude of
∇
φ
g
(
r
), the greater the produced force.
The quantity –G
∇
φ
g
(
r
) in equation 4 is termed the gravitational acceleration and is sometimes symbolized as g(r).
Thus equation 4 may be rewritten to yield the more condensed expression for gravitational force:
F
g(
r
)
=
m
o
g(
r
).
Often the magnitude of a gravitational accelerating force is measured in terms of “g’s,” or multiples of Earth’s gravitational acceleration pulling us toward Earth, which at Earth’s surface has a value of about 980 cm/s
2
.
This should not be confused with the inertial “g” symbol, which quantifies the magnitude of a mechanical accelerating force experienced by a jet pilot or rocket astronaut as inertial force resisting acceleration.
Thus, an electrogravitic acceleration of 10 g’s would signify a gravitational acceleration ten times that produced naturally at Earth’s surface.
Depending on the polarity and orientation of the applied electric field, this artificially induced gravitational acceleration may be engineered either to supplement or to counter that produced by Earth’s field.
Equation 4 may be combined with proportionality relation 3 to express the gravitational force
F
g
acting on a body (or dielectric) in terms of the product of the inertial mass mo of that body (or dielectric) and the voltage gradient,
∇
φ
e
(
r
), that spans it:
(5)
F
g
(
r
) = k m
o
∇
φ
e
(
r
).
The constant k added in here is an experimentally determined electrogravitic proportionality constant that quantifies the charge-to-mass coupling relationship.
Hopefully, future experimentation will provide a value for this constant.
Equation 5, then, mathematically expresses the electrical induction of a gravitational force.
