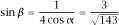Nonplussed! (5 page)

the semi-length of the cone equals 3 × 3 = 9,

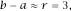
and the length of the slope equals 36

From this we can deduce from
figure 2.4
that sin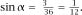
The lengths of the horizontal projections of the slopes in
figure 2.4
are each 36 cos
a
, which makes

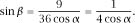
From
figure 2.6
,

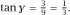
Figure 2.1.
The opening page of
Pleasure with Profit
.

Figure 2.2.
Page 12 of
Pleasure with Profit
.

Figure 2.3.
Page 13 of
Pleasure with Profit
.

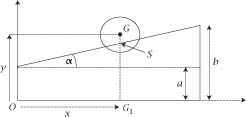
Figure 2.4.
A side view, looking at the section along
OG
₁
.

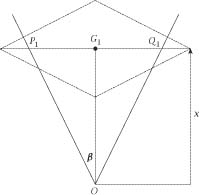
Figure 2.5.
A plan view at floor level, with the cone contacting the runners at
P
and
Q
, with
P
₁
and
Q
₁
lying directly below at floor level.

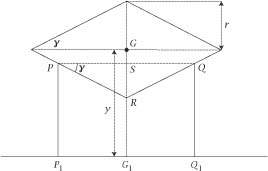
Figure 2.6.
A front view, looking from
O
.

Knowing the exact value of sin
α
enables us to use Pythagoras’s Theorem to calculate the third side of the right-angled triangle as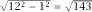
and therefore to evaluate
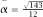and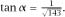

Now we have that